1.
Có 2 chiếc đồng hồ trong phòng của Cathy. Một chiếc chậm 25 phút. Hiện giờ nó đang chỉ 7:40. Chiếc thứ 2 nhanh 15 phút. Hỏi chiếc thứ 2 đang chỉ mấy giờ?
3.
Bố treo 4 chiếc khăn mặt trên một chiếc dây phơi. Bố muốn dùng càng ít chiếc kẹp càng tốt. Với 3 chiếc khăn mặt, bố cần 4 chiếc kẹp. Hỏi bố cần bao nhiêu chiếc kẹp nếu muốn treo 11 chiếc khăn mặt?

4.
Một tiết toán dài 45 phút. Peter có thể giải bất cứ bài tập nào trong sách mất 6 phút. Tuy nhiên bạn ấy cần nghỉ 1 phút sau khi giải mỗi bài toán. Hỏi Peter có thể giải bao nhiêu bài trong một tiết toán?
5.
15 bạn nhỏ đang chơi trốn tìm. Một trong số họ sẽ phải đi tìm người khác. Sau một lúc còn 7 bạn vẫn còn đang trốn. Hỏi có bao nhiêu bạn đã được tìm thấy?
6.
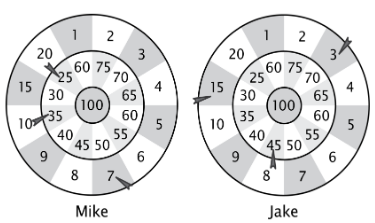
Mike và Jake đang chơi ném phi tiêu. Mỗi người được phép ném 3 lần (xem hình vẽ). Hỏi ai là người chiến thắng, và đã được bao nhiêu điểm?
7.
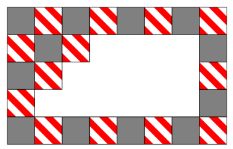
Một hoa văn hình chữ nhật trên tường được tạo với 2 loại hình vuông nhỏ: xám và sọc vằn. Một vài miếng đã bị rơi ra (xem tranh). Hỏi có bao nhiêu miếng xám bị rơi?
8.
Năm 2012 là năm nhuận, tức là sẽ có 29 ngày trong tháng Hai. Vào ngày 15 tháng 3 năm 2012, một chú vịt được 20 ngày tuổi. Hỏi chú vịt sinh ra từ vỏ trứng khi nào?
9.
Phần B - Mỗi câu đúng 4 điểm
Bạn có các miếng xếp hình chữ L, mỗi miếng bao gồm 4 hình vuông nhỏ như dưới đây:
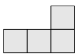
.
Hỏi có bao nhiêu hình dưới đây được tạo từ 2 miếng xếp hình trên?
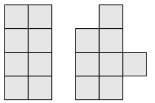
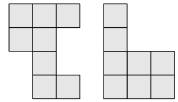
10.
Once Little Piggy planted 8 acorns. In a few years, oak trees grew from all acorns except two of them. All oak trees except two of them had their acorns. Acorns from all profilic trees except one, are very tasty. How many oaks with tasty acorns are there?
11.
In a sudoku the numbers 1, 2, 3, 4 can occur only once in each column and once in each row. In the mathematical sudoku on the picture, Patrick first writes in the results of the calculations. Then he completes the sudoku. Which number will Patrick put in the grey cell?

12.
Grandmother made 20 gingerbread biscuits for her grandchildren. She decorated them with raisins and nuts. First she decorated 15 biscuits with raisins and then 15 biscuits with nuts. At least how many biscuits were decorated both with raisins and nuts?
13.
Among Nikolay's classmates there are twice as many girls as there are boys. Which of the following numbers may be equal to the number of all children in this class?
14.
In the animal's school, three kittens, four ducklings, two goslings and several lambs are taking lessons. The teacher owl found out that all of her pupils have 44 legs altogether. How many lambs are among them?
15.
In one kangaroo family, there is a custom to name their babies by names, which consist of two letters of the word KANGAROO: the first letter is a consonant, the second letter is a vowel. How many different baby names can be composed?
16.
At a Christmas party, there was exactly one candlestick on each of the 15 tables. There were six five-branched candlesticks, and the rest were three-branched ones. How many candles had to be bought for all the candle sticks?
17.
Part C: Each correct answer is worth 5 points
The figure consists of an equilateral triangle, a square and a regular pentagon. It is built by sticks of equal length. At most many such figures can be built wit h a box of 2012 sticks of equal length ?
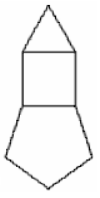
18.
Gregor forms two numbers with the digits 1, 2, 3, 4, 5 and 6. Both numbers have three digits, and each digit is used only once. Gregor adds these two numbers. What is the greatest sum Gregor can obtain ?
19.
Laura, Iggy, Val and Kate want to be in one photo together. Kate and Laura are best friends and they want to stand next to each other. Iggy wants to stand next to Laura because h e likes her. In how many possible ways can they arrange for the photo?
20.
A grasshopper wants to climb a staircase with many steps. She can make only two kinds of jumps: three steps up , or four steps down. Beginning at th e ground level, at least how many jumps will she have to make in order to take a rest on the 22
th step?

21.
Frank made a domino snake of seven tile s. He put the tiles next to each other so that the sides with the same number of dots touch ed . Originally the snake had 33 dots on its back. However, Frank's brother George took away two tiles from the snake (see the picture).
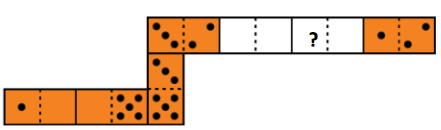
How many dots were in the place with the question mark?
23.
A rectangular paper sheet measures 192 × 84 mm. You cut the sheet along just one straight line to get two parts, one of which is a square. Then you do the same with the non - square part o f the sheet, and so on. What is the length of the side of the smallest square you can get with this procedure?
24.
In a soccer game the winner gains three points, while the loser gains zero points. If the game i s a draw, then the two teams gain one point each. A team has played 38 games and gain ed 80 points. Find the greatest possible number of games that the team lost.