3.
There are ten ducks. Five of these ducks each lay an egg every day. The other five ducks each lay an egg every second day. How many eggs in total do the ten ducks lay in a period of 10 days?
4.
The figure shows a board where each small square has an area of 4 cm2. What is the length of the thick black line?
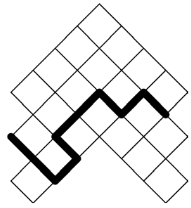
5.
The two pictures of the balanced scales show the birds Rita and Dita.

How much does Dita weigh?
6.
Which of the following fractions is smaller than 2?
7.
Each plant in John's garden has either 5 leaves only, or 2 leaves and 1 flower. In total, the plants have 6 flowers and 32 leaves.How many plants are there?

8.
Alva has 4 paper strips of the same length. She glues 2 of them together with a 10 cm overlap, and gets a strip 50 cm long.
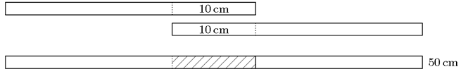
With the other two paper strips, she wants to make a 56 cm long strip.How long should the overlap be?
9.
Nine flowers are planted in a row, same distance apart between any two adjacent flowers. The distance between the first and the third flower is 60 cm. What is the distance between the first and the last flower?
10.
Lisa has 90 marbles. Anna has 10 more marbles than Lisa but 50 less marbles than Olle. How many marbles do they have together?
11.
Part B: Each correct answer is worth 4 points11
In the picture, the rectangle ABCD is constructed from four identical rectangles. If the length of the segment BC is 1 cm, what is the length of the segment AB?

12.
Lucy and her mother were both born in January. On March 29, 2015, Lucy adds the year of her birth, the year of her mother’s birth, her age, and her mother’s age. What is the result?
13.
A rectangle has an area of 12 cm2. Its sides are of integer lengths in centimetres. Which of the following values could be the perimeter of the rectangle?
14.
Each of the 9 line segments in the figure is to becoloured either blue, green or red. The three sides of each triangle are to have three different colours. Three of the line segments have already been coloured, as shown. What colour can the line segment marked with x have?

15.
Carla wants to fold a cube from a paper net. By mistake she drew 7 squares on her sheet instead of 6 squares. Which square(s) can she remove so that the figure remains connected and Carla can fold a cube from it?
16.
The number 100 is multiplied either by 2 or by 3, then the result is increased either by 1 or by 2, and then the new result is divided either by 3 or by 4.If the final result is a natural number, what is this final result?
17.
In a four-digit number
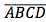
, the digits A, B, C and D are in increasing order from left to right. What is the largest possible difference
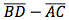
of the two-digit numbers
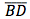
and
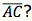
18.
Kangarina discovered that someone had eaten her jar of honey. She suspected one of her four neighbours: the antelope Anty, the bear Beary, the fox Foxy orthe lion Leo. Anty stated that Beary ate the honey. Beary stated that it was Leo. Foxy and Leo denied eating the honey. Who ate the honey if only one of them is telling the truth?
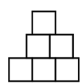
19.
Tom used 6 squares with sides of length 1 to form the shape in the picture. What is the perimeter of the shape?
20.
Part C: Each correct answer is worth 5 points
Square paper sheets of two sizes are needed for an art project. How many of the smaller sheets are needed to cover one of the larger sheets if it is known that the perimeter of one of the larger sheets is eight times as great as the perimeter of one of the smaller sheets?
21.
On Jump Street, there are 9 houses in a row. At least one person lives in each house. Any two neighbouring houses together are inhabited by at most six people. What is the largest number of people that could be living on Jump Street?
22.
In a bag there are 3 green apples, 5 yellow apples, 7 green pears and 2 yellow pears. Simon randomly is taking fruits out of the bag one by one. How many fruits must he take out in order to be certain that he has at least one apple and one pear of the same colour?
23.
Two cats, Tom and Bob, caught together 42 mice in three days. Each day, Tom caught twice as many mice as in the previous day, while Bob caught two more mice than in the previous day. Nonetheless, both cats caught the same number of mice for the three days. How many mice did Tom and Bob catch together during the first day?
24.
Five children were standing in a line and holding 37 balloons in total. All children who were to the right of Andrew had together 14 balloons; all children standing to the right of Bob had together 32 balloons; the ones to the right of Clara – a total of 20 balloons, and to the right of Danny – 8 balloons. How many balloons did Clara hold?
25.
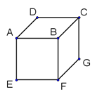
Mary wrote a number on each face of a cube. Then, for each vertex, she added the numbers on the three faces sharing that vertex (for example, for vertex B she added the numbers on faces BCDA, BAEF and BFGC). The numbers computed by Mary for vertices C, D and E were 14, 16 and 24, respectively. What number did she compute for vertex F?
26.
A train has 12 coaches. Each coach has the same number of compartments. Mike is travelling in the third coach and in the 18th compartment from the engine. Jane sat in the 7th coach in the 50th compartment from the engine. How many compartments are there in each coach?
27.
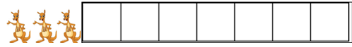
In how many ways can you place the three kangaroos in three different cells, out of the seven cells below, so that no two kangaroos are neighbours?
28.
Four points lie on a line. The distances between them are, in increasing order: 2, 3, k, 11, 12, 14. What is the value of k?
29.
Basil used small cubes with side 1 to construct a cube with side 4. After that, he painted 3 faces of the big cube red and the other 3 faces blue. After he finished, there was no small cube with 3 red faces. How many small cubes have both red and blue faces?